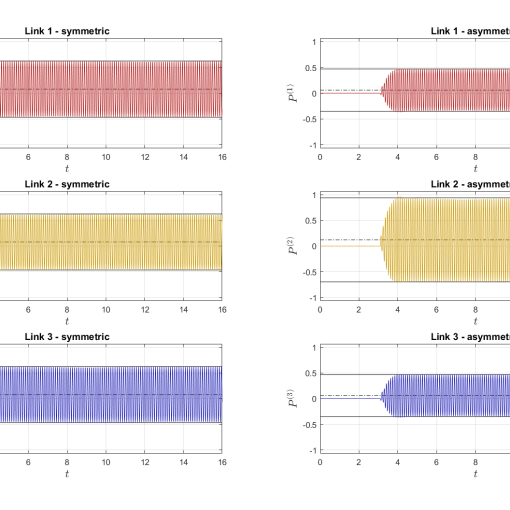
A fully analytical controller design is proposed to tackle a periodic control problem for stable linear systems with an input delay. Applying the internal model control scheme, the controller design reduces to designing a filter, which is done through placement of poles and zeros. The zeros are placed to compensate for the harmonics and to gain the filter properness. For placing the poles, a quasi-optimal procedure is proposed utilizing the standard LQR method. Supposing high-dimensionality of the filter due to targeting large number of harmonics, the design as well as controller implementation is performed over a state space representation. A thorough experimental case study is included to demonstrate both the practical feasibility and effectiveness of the proposed control design. The experimental validation is performed on a physical system where the goal is to reject periodic vibrations acting on a mass-spring-damper setup where the sensor and the actuator are non-collocated.
You may also like
Wim Michiels1, Senior Member, IEEE, Tomas Vyhlidal2, Karel Kraus3, Zbynek Sika3 1Department of Computer Science, KU Leuven, Leuven, 3001, Belgium, e-mail: wim.michiels@cs.kuleuven.be2Department […]
Fig. 1 Experiment without inverse shaper References [1] Dan Pilbauer, Wim Michiels, Tomáš Vyhlídal, Martin Hromčík, Mixed-sensitivity design of a dynamic controller […]
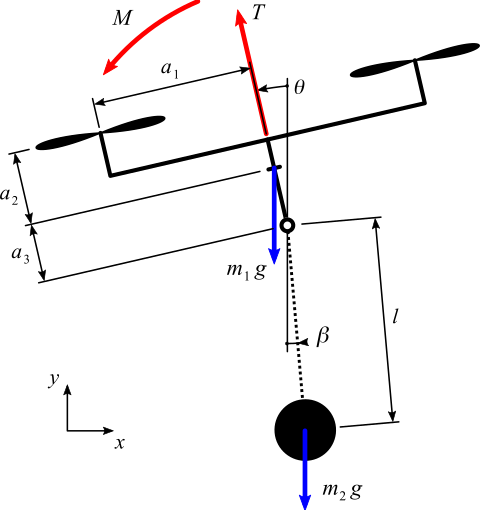
A control design and numerical study is presented for the problem of maneuvering a quadcopter with suspended load. An inverse shaper with a […]
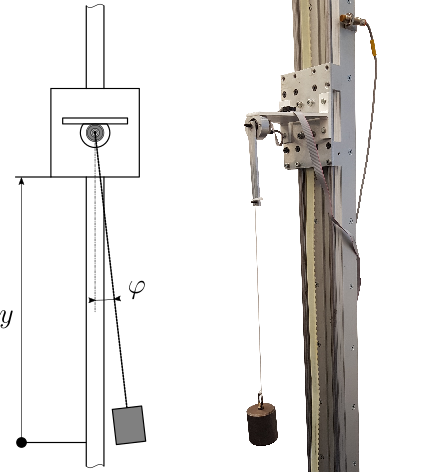
Solution of a case study problem of suspended payload sway damping by moving a pivot base in vertical direction is presented. Unlike […]