Authors: Tarunraj Singh, Tomáš Vyhlídal, Jaroslav Bušek
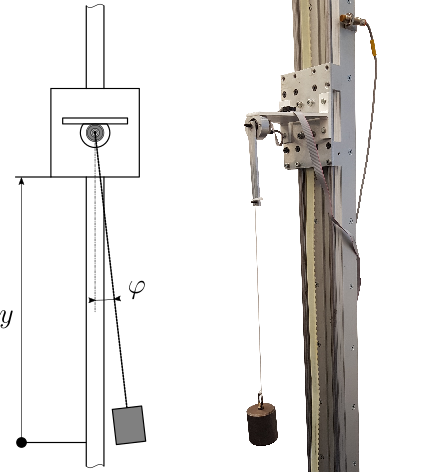
The focus of this paper is on the design of distributed delay multi-mode input shapers which emphasize smooth transients and reveal a favorable placement of the shaper zeros. The shaper is linearly parameterized using Bernstein polynomials as the kernel functions. The nonnegative magnitude of the Bernstein polynomials over the support of the shaper allows the formulation of convex constraints to design the shaper parameters, whereby the jerk constraints can be easily accommodated. The asymptotic properties of the infinite chain of zeros of the shaper are prescribed by using linear constraints. Including the shaper structure and robustness constraints, the design reduces to solving a feasibility problem. The shaper design and its performance are demonstrated, both numerically and experimentally, on a model of a helicopter with a suspended load featuring two flexible modes to be compensated for.